CFDにおけるFEMとFVM
FEM and FVM in CFD
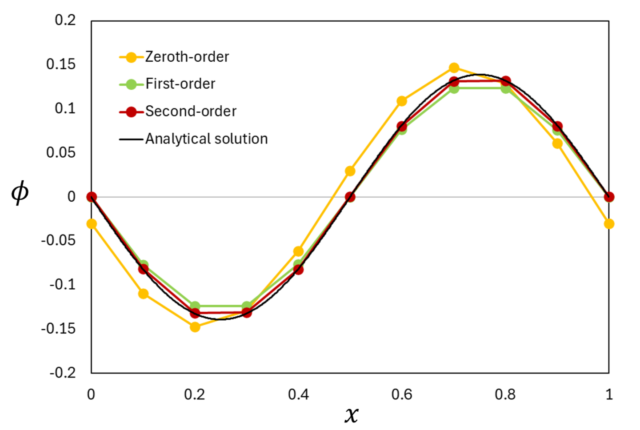
構造解析では有限要素法、流体解析では有限体積法。偏微分方程式を離散的に解く手法という意味ではどちらも共通しているのに、構造と流体ではなぜ違う手法が一般的なのでしょうか?本記事では、流体解析における有限要素法と有限体積法を比較して「なぜCFDでは有限体積法が一般的になったか」の理由を垣間見ていきます。
Structural analysis uses the finite element method, while fluid analysis uses the finite volume method. Why are different methods commonly used for structure and fluid analysis, even though both are the same in the sense that they are methods for solving partial differential equations discretely? In this article, we compare the finite element method and the finite volume method in fluid analysis and give a glimpse into the reason “why the finite volume method became common in CFD."

古澤善克
Yoshikatsu Furusawa
2024,08,23 2024,08,23