皆さんは Grasshopper で最適化をしたことがありますか?特に Tunny をつかって最適化をしたことがありますか?
その時にうまく最適化できないなと思ったことはないでしょうか。
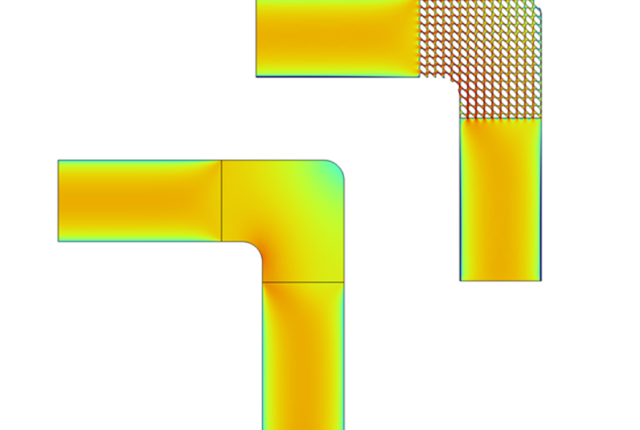
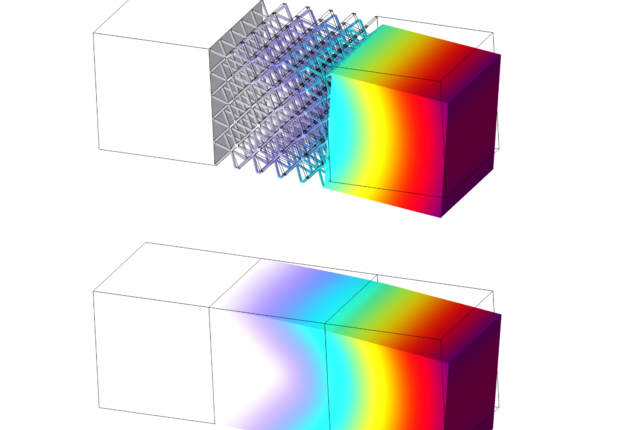
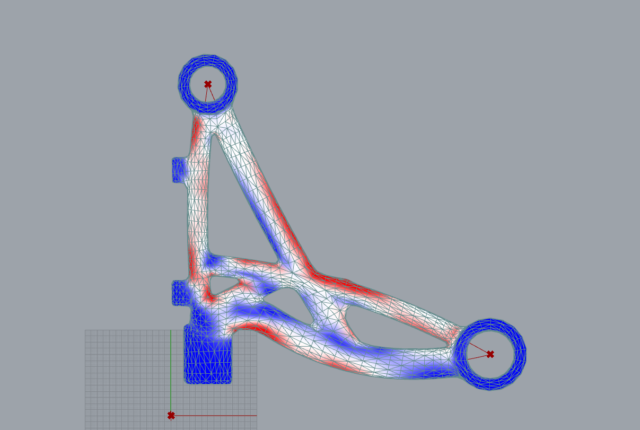
Tunny が採用しているブラックボックス最適化と呼ばれる部類の最適化は感度を使用した最適化(例えば位相最適化)に対して収束が遅いため、適切な最適化設定でなかったり、対象の問題が適切でないと、ランダムより少し良い結果が得られる程度のものになってしまいます。
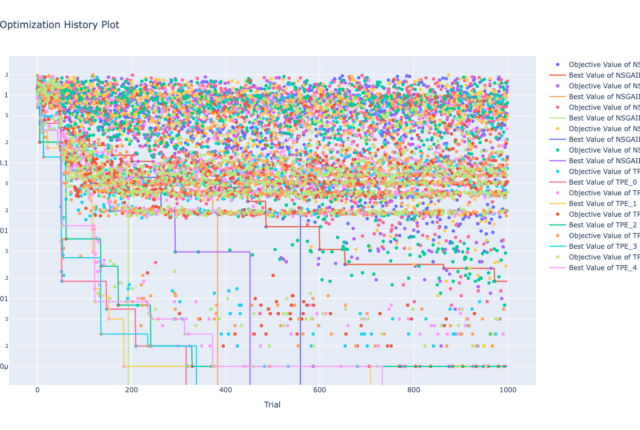
今回は Tunny を使って、最適化設定の違いによる結果の違いを見ていきましょう。
Have you ever performed optimization in Grasshopper? Specifically, have you used Tunny for optimization?
During that time, did you ever feel that the optimization was not working well?
The category of optimization called black-box optimization, which Tunny uses, has slower convergence compared to sensitivity-based optimization (such as topology optimization). If the settings are not properly configured or the problem is not suitable, the result may end up being just slightly better than random.
In this article, let's look at the optimization results using Tunny with different settings.